LIGHT ABSORPTION AND FATE OF EXCITATION ENERGY:
Franck-Condon Principle
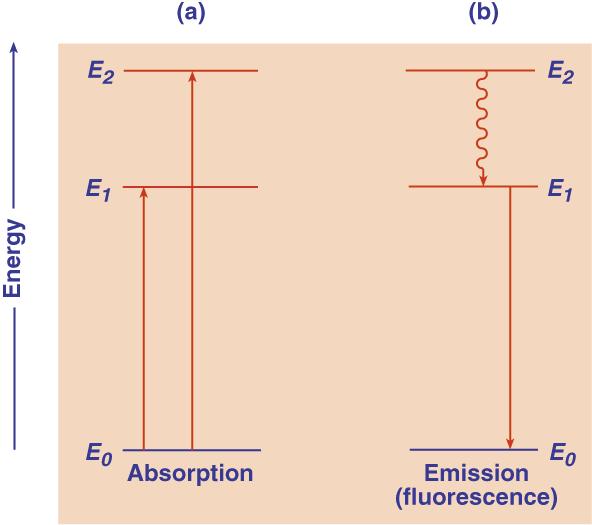
What happens to an atom or molecule that absorbs a photon? When light is absorbed, each quantum is taken up by a single atom or molecule and the whole energy of the quantum is communicated to it. The absorbing atom or molecule is thus excited, that is, lifted from its normal state of lowest energy (highest stability) to an excited, energy-rich state. According to Niel Bohr's theory of atomic and molecular structure, an atom or molecule can exist only in a series of discrete states of electronic energy. This is represented below.

Ground state (E
0) and two excited states (E1, E2) of a molecule (vibrational and rotational levels are not shown).The energy levels are indicated, as they usually are in spectroscopy, by properly spaced horizontal lines. The lowest line represents the ground state, E0, of the atom or molecule in which it exists in the absence of external activation. The higher lines (E1 and E2) represent excited electronic states--the only kind of excited states possible in an atom. In a diatomic or polyatomic molecule, one or several series of (also quantized) vibrational and rotational states are superimposed on each electronic state. If the molecule is complex, the various vibrational and rotational states lie very close together. The sharp absorption (and emission) lines of atoms, or structured bands of simple molecules, are replaced by broad, continuous bands. The absorption lines (or bands) are represented, in schemes of this type, by arrows directed upwards and the emission lines or bands by arrows directed downwards (see the figure shown above). The energy of the quanta of emission or absorption is proportional to the lengths of the arrows. An atom or molecule can absorb only energy quanta corresponding to the distances between the permitted energy states. Once it is excited, say to state E2, it can release energy represented by downward arrows, leading from E2 to a lower energy state E1. The wavy arrow in the above figure (from E2 to E1 ) relates to another, radiationless way in which a transition can occur by energy loss to surrounding molecules, or by its "internal conversion" into vibrational energy of the excited molecule. The fall from E2 to E1 in one big jump is fluorescence. In fluorescence, light absorption leading, say, from E0 to E1 is reversed by light emission leading from E1 to E0. When a photon is absorbed, the molecule usually is not merely transferred into an excited electronic state, but also acquires some vibrational energy. According to the so-called Franck-Condon principle**, the absorption of a photon is a practically instantaneous process, since it involves only the rearrangement of practically inertia-free electrons. James Frank recognized the obvious: the nuclei are enormously heavy as compared to the electrons. Thus, during light absorption, that occurs in femtoseconds, electrons can move, not the nuclei. The much heavier atomic nuclei have no time to readjust themselves during the absorption act, but have to do it after it is over, and this readjustment brings them into vibrations. This is best illustrated by potential energy diagrams, such as that shown below. It is an expanded energy level diagram, with the abscissa acquiring the meaning of distance between the nuclei, rxy. The two potential curves show the potential energy of the molecule as a function of this distance for two electronic states, a ground state and an excited state. Excitation is represented, according to the Franck-Condon principle, by a vertical arrow (A). This arrow hits the upper curve, except for very special cases, not in its lowest point, corresponding to a nonvibrating state, but somewhere higher. This means that the molecule finds itself, after the absorption act, in a nonequilibrium state and begins to vibrate like a spring. This vibration is described, in the figure, by the molecule running down, up, down again, etc., along the upper potential curve, like a pendulum. The periods of these vibrations are of the order of 10-13, or 10-12 seconds. Since the usual lifetimes of excited electronic states are of the order of 10-9 s, there is enough time during the excitation period for many thousands of vibrations. During this time much if not all of the extra vibrational energy. is lost by energy exchange (temperature equalization) with the medium. The molecule, while it remains extremely "hot" as far as its electronic state is concerned, thus acquires the ambient "vibrational temperature." Fluorescence, when it comes, originates from near the bottom of the upper potential curve, and follows a vertical arrow down (F), until it strikes the lower potential curve. Again, it does not hit it in its deepest point, so that some excitation energy becomes converted into vibrational energy. The cycle absorption-emission thus contains two periods of energy dissipation. Because of this, the fluorescence arrow (F) is always shorter (that is, the fluorescence frequency is lower) than that of absorption (A). In other words, the wavelengths of the fluorescence band are longer than of the absorption band. This displacement of fluorescence bands towards the longer wavelengths compared to the absorption bands (Stokes' shift) was a long-established experimental fact before the Franck-Condon principle provided its interpretation. Obviously, the extent of the shift depends on the difference between the two potential curves.
---------------------------------------------------------------------------------------------------------
**How did it become known as .Franck-Condon Principle?
[From Govindjee (1999) In "Photochemistry of Carotenoids" ,edited by H.Frank et al., Kluwer Academic, Dordrecht,
The Netherlands.]
A historical suggestion was that of James Franck (1925, who had shared, with Hertz, the 1925 Nobel Prize in Physics for the experimental verification of the quantum theory). He argued simply that because of the large masses of the nuclei in a molecule, their relative momentum cannot be directly affected by an electronic transition, so that those transitions will be most likely that conform most closely to a Principle. The nuclei do not move during an electronic transition. Thus, on a diagram of energy (ordinate) versus distance between the nucleii of a diatomic molecule (abscissa), this transition is vertical promoting the electron from the lowest vibrational state of a molecule in the ground state to a higher vibrational state of the excited state (e.g. S1 or S2) of the molecule. The molecule in the excited state then dissipates immediately (within 10 to 100 fs) some energy as heat and the electron reaches the lowest vibrational level of the excited state. When the molecule relaxes to the ground state giving off light (fluorescence), it generally occurs at longer wavelength than the absorbing wavelength (Rotverschiebung, the red shift, Franck, 1927). The Franck-Condon principle, then, explains the observed red shift (Stokes, 1852) of the fluorescence spectrum from the absorption spectrum. The history of how the principle became known as the Franck-Condon principle was beautifully presented by Condon (1947). The original idea is in a paper at a Faraday Society meeting in London by Franck (1925); the proofs of this paper were sent to his student Hertha Sponer, who was then at the University of California at Berkeley on an International Education Board Fellowship. She generously shared the proofs with Condon; he was able to generalize Franck's ideas (Condon, 1926). Condon (1947) states: "This work was all done in a few days. Doctor Sponer showed me Franck's paper one afternoon, and a week later all the quantitative work for my 1926 paper was done."
------------------------------------------------------------------------------------------------------------
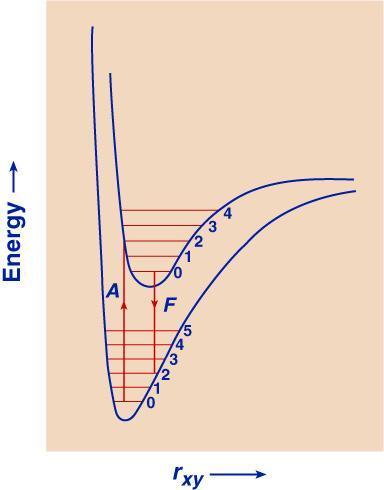
Frank-Condon Principle.
Potential energy curves for the ground state and an excited state of a diatomic molecule.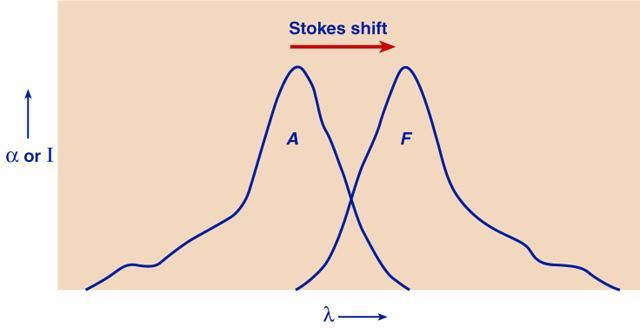
The Stokes' shift
(displacement of fluorescence band compared to the absorption band of a molecule). Approximate mirror symmetry of the two bands exists when the shapes of the potential curves in the ground state and the excited state are similar.